Racing Card Derby (RCD) is a relatively new table game that uses an innovative electronic interface to dress up what is otherwise a very simple idea – betting on the suit of a dealt card. The novelty of this conglomeration has yielded RCD placements both in the U.S. and internationally. From what I can tell, if RCD is offered according to best practices, there is no clear avenue to advantage play. However, a fundamental issue with RCD's design may encourage a specific change in procedure that is outside the game's best practices. As we will see, if such a change is made, things can go very wrong.
RCD is meant to be dealt from a single deck of cards, shuffled between rounds. To play a round, the cards are dealt, one at a time, and the suit of the exposed card is recorded. Cards continue to be dealt and exposed until one of the suits appears four times. The first suit to have four dealt is referred to as the winning suit, or simply, the “Winner.” Note that at least 4 cards and at most 13 cards must be dealt to determine the winning suit (exercise). The player can also wager on the color of the winning suit (red or black).
To complete the round, two additional suits must be dealt. First, additional cards are dealt until a suit other than the winning one appears. The suit of that card gives the 2nd-place suit. Then, additional cards are dealt until a suit other than the winning or 2nd-place suit appears. That is the 3rd -place suit. There are additional wagers based on these subsequent cards, called the “Exact Trifecta” and “Exact Quintella”.
Altogether, in a single round of RCD, as few as 6 cards and as many as 33 cards may be dealt (exercise). I simulated one hundred million (100,000,000) rounds and found that the average number of cards dealt per round is 12.11.
This analysis focuses solely on a player who wagers on the winning suit (the “Winner” bet). Clearly, true odds are 3-to-1. To generate a house edge the designers added a "protest card" rule. The payout to the player is reduced if the Two of Spades (2S) is dealt in the process of determining the winning suit. Here are the payouts:
-
Player picks winning suit and no 2S is dealt: pays 3-to-1.
-
Player picks winning suit and 2S is dealt: pays 2-to-1.
-
Otherwise, the player loses.
Clearly, the house edge will be slightly higher for a wager on Spades than if the player wagers on Clubs, Hearts or Diamonds. This is because a winning Spades wager is much more likely to have the 2S occur than a win in any other suit.
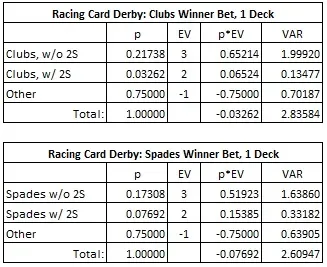
The first table below gives the combinatorial analysis for wagering on Clubs. This analysis also works for a wager on Diamonds or Hearts. The second table below gives the combinatorial analysis for a wager on Spades:
In particular,
-
The house edge for a Clubs, Diamonds or Hearts Winner Bet is 3.262%.
-
The standard deviation for a Clubs, Diamonds or Hearts Winner Bet is 1.684.
-
The house edge for a Spades Winner Bet is 7.692%.
-
The standard deviation for a Spades Winner Bet is 1.615.
This is a very simple game – pick a suit, draw some cards, see if your suit wins. The key element of RCD that makes it attractive is the game’s interface with the player. The game designers decided to create an electronic video screen that simulates a horse race, where the horses move towards the finish line by virtue of the sequence of suits drawn. This video display requires the use of an optical device built into the table that reads the suit of each card as it is dealt. The best way to understand how this occurs is to watch the following YouTube video demonstration of the game:
In particular observe that the cards are dealt from a one-deck shoe and slid over the reader. Note also the time spent inserting/removing the cards from the shoe, and well as the insufficient shuffle demonstrated in the video.
Assuming players are playing the game in large enough numbers so that the casino wants to keep the game, the game's profitability comes down to two elements: house edge and game pace. A word about each.
With a house edge of 3.262% (higher for a bet on Spades), the edge is lower than roulette as well as many other novelty games. Without a strategic decision by the player, there is no way the player can make an error that increases the house edge. Like roulette, the edge is the edge, and in this case the edge may be too low.
More problematic, however, is game pace. Each round requires from 6 to 33 cards to be dealt. There is also the graphical display that has to complete its audio/visual cycle. Multiple wagering opportunities may make reconciling winning wagers time-consuming. There is frequent hand shuffling. Using a shoe to deal a single-deck will also slow down the game.
Because the house edge cannot be adjusted without a complete redesign of the rules, the main solution to increasing profitability is to address the game pace issue. The most natural solution to game pace is to increase the number of decks and to deal multiple rounds between shuffles. By minimizing shuffle time, and transfer time to/from the shoe, the game will generate significantly more wagering opportunities per hour. With an eye towards both game protection and optimizing game pace, the most natural number of decks to use would be eight (8). Using an eight-deck shoe, an intuitively safe placement of the cut card, similar to what is used in blackjack, would be two decks from the end (cut card placed at 312 cards).
Here is the question I will answer in the rest of this blog article: If RCD is dealt from an eight-deck shoe, with the cut card placed at 312 cards (two decks from the end), what is the vulnerability of the Winner Bet in RCD to card counting?
I first consider the case of card counting Clubs. The following table gives the combinatorial analysis of a Winner Bet placed on Clubs when RCD is dealt from an eight-deck shoe:
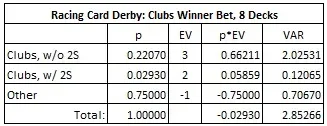
Note that the house edge has been reduced from 3.262% to 2.930%. What the casino gained in game pace, they may be losing right back in reduced house edge.
The following table gives the effect-of-removals (EOR) and a candidate counting system:
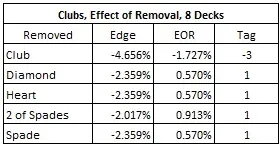
This counting system is as easy as it gets: Clubs count -3, all others count +1.
Here are the results of a simulation of one hundred million (100,000,000) eight-deck shoes of RCD, using the count above to make wagers on the Winner Bet on Clubs:
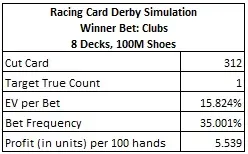
If a solo AP was wagering $100 on Clubs whenever he had the edge, and making no other wagers, then he would earn $553.90 per 100 hands.
As far as advantage play is concerned, there is no difference between Winner Bets on Clubs, Diamonds or Hearts. A similar card counting system can be used on each. Both the EV per Bet and Bet Frequency will be the same for these other wagers, leading to a profit of 5.539 units on each suit-wager per 100 hands. The problem is that a single AP will find it a challenge to keep three different counts. This is where a team of three APs comes in, leading to a nine-fold compounding of casino-loss.
Suppose:
-
RCD dealt from an eight-deck shoe, with the cut card placed two decks from the end.
-
A team of three APs.
-
One member of the team counts Clubs, one counts Diamonds and one counts Hearts.
-
Each team member wagers on his suit when the count indicates.
-
The other APs watch each other, and whenever a team member makes a wager on a suit, the other members of the team also wager on that suit.
Then:
-
Each member of the team will earn 3 x (5.539) = 16.617 units per 100 hands.
-
The team will earn 3 x (16.617) = 49.851 units per 100 hands.
If the APs are wagering $100 each, then the team will win approximately $4985.10 per 100 hands.
The Winner Bet on Spades is also countable, however it is not nearly as lucrative due to the negative effect of the 2S on the house edge. The following table gives the combinatorial analysis of a Winner Bet placed on Spades when RCD is dealt from an eight-deck shoe:
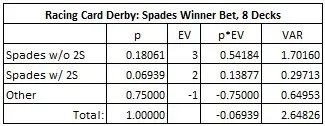
The following table gives the effect-of-removals (EOR) and a candidate counting system:
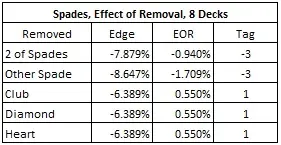
Again, this is a trivial card counting system: Spades = -3, all others = +1.
Here are the results of a simulation of one hundred million (100,000,000) eight-deck shoes of RCD, using the count above to make wagers on the Winner Bet on Spades:

If a solo AP was wagering $100 on Spades whenever he had the edge, and making no other wagers, then he would earn $265.20 per 100 hands.
If we add a fourth AP to the team whose job it is to count Spades:
-
RCD dealt from an eight-deck shoe, with the cut card placed two decks from the end.
-
A team of four APs.
-
One member of the team counts Clubs, one counts Diamonds, one counts Hearts and one counts Spades.
-
Each team member wagers on his suit when the count indicates.
-
The other APs watch each other, and whenever a team member makes a wager on a suit, the other members of the team also wager on that suit.
Then:
-
Each member of the team will earn 3 x (5.539) + 2.652 = 19.269 units per 100 hands.
-
The team will earn 4 x (19.269) = 77.076 units per 100 hands.
If the APs are wagering $100 each, then the team will win approximately $7707.60 per 100 hands.
By keeping track of these four suits, there may be a number of additional wagering opportunities for the team of APs. For example, if both Diamonds and Hearts are indicated, then wagering on “Red” is also implied. Also, the Quinella and Trifecta may be vulnerable using the combination of these four counts. I have not analyzed these or other counting possibilities, however it would come as no surprise if a team of four APs could earn over $10,000 per 100 hands with $100 wagers.
Here are my game protection recommendations for guarding RCD from card counting. Essentially, they amount to avoiding this nightmare scenario to begin with:
-
Under no circumstances should multiple rounds of RCD be dealt between shuffles. In particular, a multi-deck shoe should never be used.
-
I am also concerned that even if dealt from a continuous shuffle machine, RCD will be vulnerable to card counting due to “latency” (the time it takes for a used card to get re-inserted into the mix). I haven’t analyzed this, however.
-
When dealing RCD from a single deck, use a rigorous shuffle or an automatic shuffler. The ability to track the 2S can lead to a player edge in the single deck version.
The lesson is that a game's design, by itself, may not make the game vulnerable to advantage play. However, if an aspect of the design apparently inhibits the game’s profitability, casino management may take it upon themselves to “fix” the game. In the worst case scenario, such a fix may become an opportunity for the AP of the most unimaginable sort.


